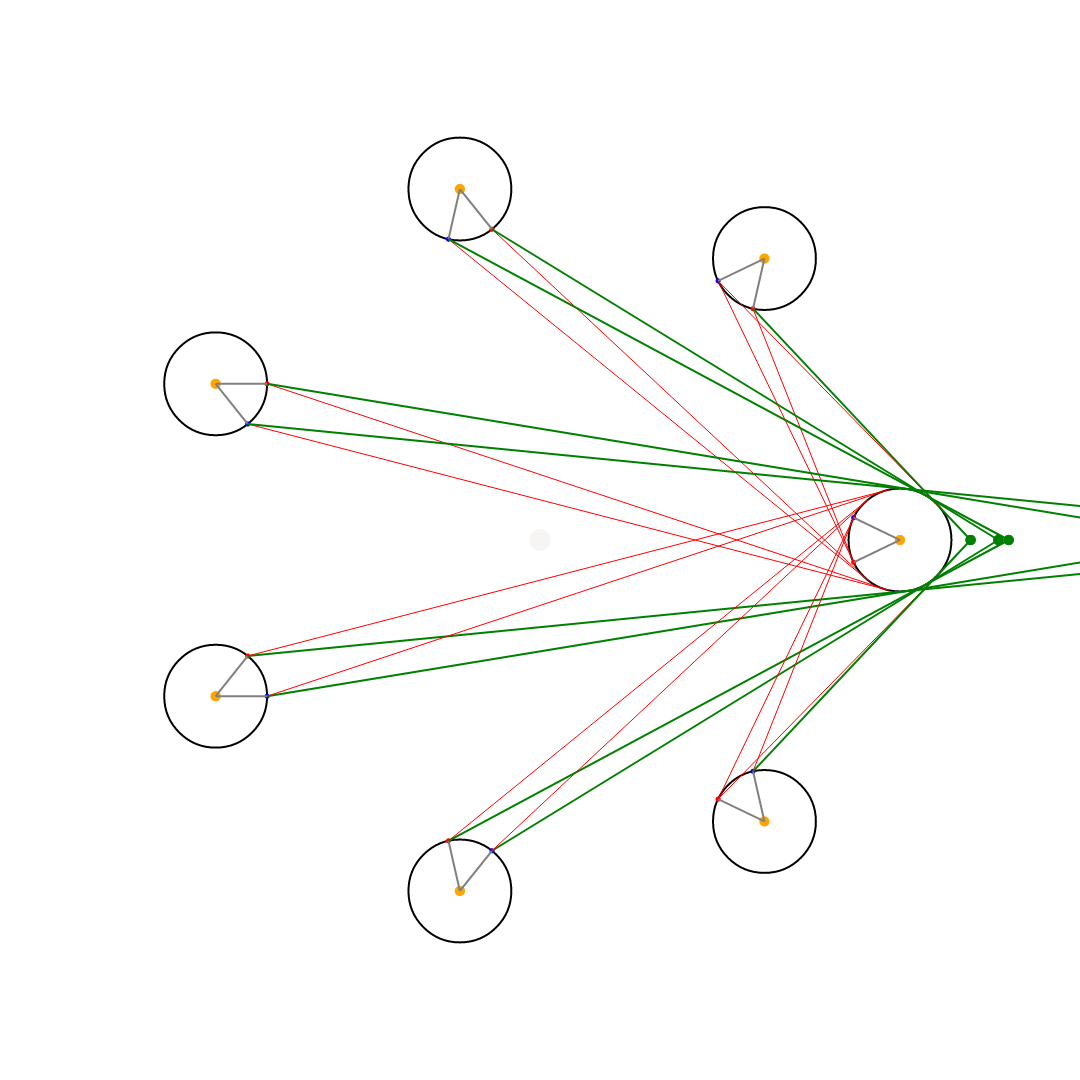
A Lighthouse Illumination Problem

This paper discusses a problem that consists of $n$ "lighthouses" which are circles with radius 1, placed around a common center, equidistant at $n$ units away from the placement center. Consecutive lighthouses are separated by the same angle: $360^\circ/n$ which we denote as $\alpha$. Each lighthouse "illuminates" facing towards the placement center with the same angle $\alpha$, also called "Illumination Angle" in this case. As for the light source itself, there are two variations: a single point light source at the center of each lighthouse and point light sources on the arc seen by the illumination angle for each lighthouse. The problem: what is the total dark (not illuminated) area for a given number of lighthouses, and as the number of lighthouses approach infinity? We show that by definition of the problem, neighbor lighthouses do not overlap or be tangent to each other. We propose a solution for the center point light source case and discuss several small cases of $n$ for the arc light source case.

PDF Abstract